In mathematics we utilise quadratics and it can represent real life situations such as describing shapes, to show how a product is increasing or decreasing in sales. It has a U shaped curve known as a parabola, it can vary in the being of the parabola are proportional to changes in the square of that parabola’s width. General formula for quadratics is y = ax2 + bx + c but quadratics can also be written as y = a(x – h)2 + k which helps identify the turning point of any equation. Parabolas can both have y-intercept and x-intercept. The y-intercept could be recognised by when the parabola intercepts at the y-axis and the x-intercept could be seen by when the parabola intercepts at the x-axis. In quadratics, a parabola can have 2, 1 and 0 x-intercepts. It can have 2 when both lines intercepts at the x-axis, it can have 1 when the centre intercepts and it can have 0 when there is nothing intercepting the x-axis.
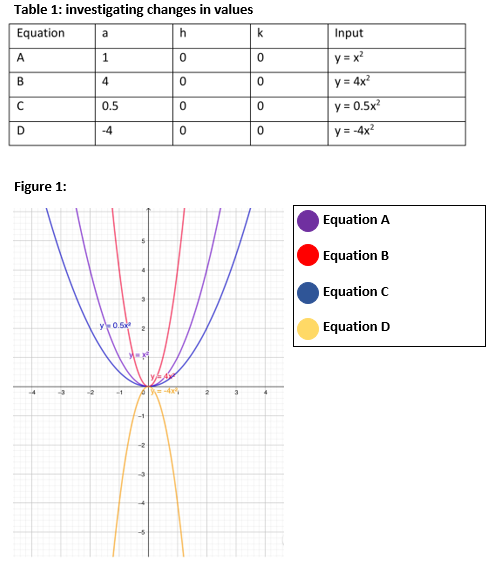
For equations A-D they all have the same turning point which is (0,0). However, for equations A-C turning points are at minimum because the a value is positive while equation D turning points are at maximum because the a-value is negative. Since the turning point is (0,0) the axis of symmetry is vertically aligned with it and therefore the quadratics is symmetrical as the left hand side of the graph has the same appearance as the right hand side. Since the values of h and k are 0, all the graphs does not have vertical nor horizontal shifts. They are merely the same position as equation A but has different dilations that change the width of the graph.
In comparison to equation A, equation B’s width is narrower because the a value is 4 which is greater than the a value in equation A. In equation C, the width is larger than equation A even though the a-value is smaller. Equation D is narrower than equation A as well as it is flipped because of the negative a value. In addition, equation D has the same shape as equation B but it is mirrored reflection due to the negative a-value. The a-value determines every width of the graph, if the value of a is less than 1 then the graph increases its width because equation y=ax2 increases slower and if the value of a is greater then the graph decreases its width because equation y=ax2 increases faster. By observing the trends within these graphs it can be deduced that in order to get a wider graph than equation A the a-value between 0 and 1. If the a-value is less than 0 it becomes a maximum parabola.
As stated in the previous task, the turning point of equation A was (0,0). As the equation of E and F are in written in turning point form, the x-coordinate can be depicted as the h-value and the y coordinate can be depicted as the k-value. Hence the turning point of equation E is (-4,5) and for equation F the turning point is (5.4,-4.3). It is evident that these h and k values shifts the graph from the equation A. As equation E is shifted horizontally to the left of equation A because the value of h is negative where as for equation F it is shifted horizontally to the right. Therefore, a negative h value would shift a graph to the left and a positive h value would shift a graph to the right. Equation E has a positive k-value which resulted the graph to shift vertically upwards where as equation F has a negative k-value which shifted the graph downwards. Therefore, a positive k-value will shift graphs up and a negative k-value will shift graphs down.
These equations with h and k values allowed them to have y-intercepts and x-intercepts unlike equation A that has y-intercepts and x-intercepts however they are placed at the origin because the h and k values are not present.
Various values can change a shape of a quadratic. When the equation is written in a turning point form, the a-value alters the width of the parabola, the h-value horizontally shifts the parabola left or right and the k-value vertically shifts the parabola up or down.